Green's Theorem and the Planimeter

I am going to start by saying I do not find Green’s theorem very intuitive. It is a magnificently powerful theorem. It has been proven, so it must be true, but unlike many other theorems, I do not feel it comes naturally. (I have mentioned this before!) But I think that is because it does not start with our normal [latex]x[/latex]-[latex]y[/latex] Cartesian plane.

There is a really good discussion of this on the Khan Academy website, which is the best explanation I have seen. The gist of it is that the relationship of a closed curve to the area it encloses is analogous to the relationship of a curve and the area beneath that curve from freshman calculus. Having such a relationship is powerful because we can use it to find the area enclosed in any two-dimensional shape using a calculation on the length of the line around it.

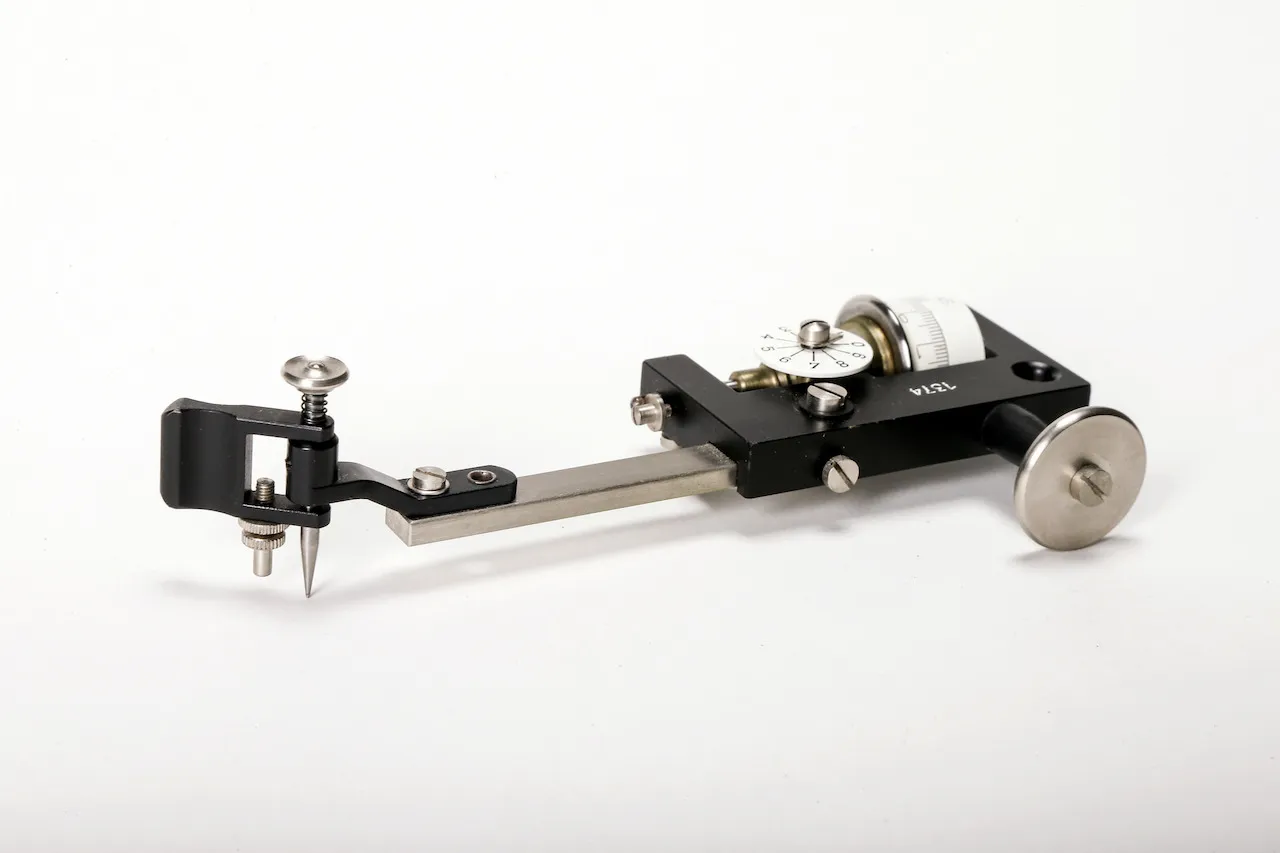
That is where the planimeter comes in. The planimeter is a device to measure the line circumscribing a shape. It also performs the calculation. The process is beautiful to watch and you can see an example here:

Integrating with a planimeter

You can use a planimeter to graph antiderivatives. Here I do antiderivatives of x, sin x, 1/x, and e^x. This is a bonus episode of my video series about calc...